The amplitude of a simple harmonic oscillator is A and speed at the mean position is v0. The speed of the oscillator at the position x = A√3 is:
1. 2v0√3
2. √2v03
3. 23v0
4. √2v0√3
The initial phase of the particle executing SHM with y = 4 sin ωt + 3 cos ωt is
1. 53°
2. 37°
3. 90°
4. 45°
A spring-block system is brought from the Earth's surface to deep inside the mine. Its period of oscillation will:
| 1. | increase |
| 2. | decrease |
| 3. | remain the same |
| 4. | may increase or decrease depending on the mass of the block |
A particle executes S.H.M with amplitude A. If the time taken by the particle to travel from -A to A/2 is 4 seconds, its time period is
1. 4s
2. 8s
3. 12 s
4. 18 s
Two simple harmonic motions are represented by y1 = 6 sin(2πt + π3) and y2 = 3(sin 2πt + cos 2πt). The ratio of their amplitudes is
1. √2
2. 23
3. 12
4. 2√2
A disc executes S.H.M. about the axis XX' in the plane of the disc as shown in the figure. Its time period of oscillation is:
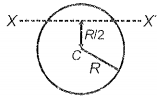
1. π√6Rg
2. 2π√Rg
3. 2π√2Rg
4. π√3R2g
A spring-block system shown in the figure oscillates with a certain time period. If charge q is given to the block and a uniform field E is switched on, then its time period of oscillation is:
1. increases
2. decreases
3. may increase or decrease
4. remains the same
The period of oscillation of the spring block system shown in the figure is: (assume pulleys and spring to be ideal)
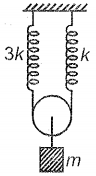
1. 2π√m3k
2. 2π√4m3k
3. 2π√3m4k
4. 2π√mk
The graph between velocity and acceleration of a particle executing S.H.M. can be
1. A circle
2. An ellipse
3. A straight line
4. Both (1) & (2)
The potential energy of a particle of mass m executing SHM is given by U = A(1 - cos2x), where x is the instantaneous displacement of the particle. The time period of oscillation is
1. π√mA
2. 2π√mA
3. π√m2A
4. 2π√m2A







