Two simple harmonic motions are represented by y1 = 6 sin(2πt + π3) and y2 = 3(sin 2πt + cos 2πt). The ratio of their amplitudes is
1. √2
2. 23
3. 12
4. 2√2
A disc executes S.H.M. about the axis XX' in the plane of the disc as shown in the figure. Its time period of oscillation is:
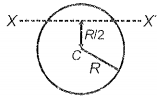
1. π√6Rg
2. 2π√Rg
3. 2π√2Rg
4. π√3R2g
A spring-block system shown in the figure oscillates with a certain time period. If charge q is given to the block and a uniform field E is switched on, then its time period of oscillation is:
1. increases
2. decreases
3. may increase or decrease
4. remains the same
The period of oscillation of the spring block system shown in the figure is: (assume pulleys and spring to be ideal)
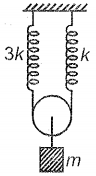
1. 2π√m3k
2. 2π√4m3k
3. 2π√3m4k
4. 2π√mk
The graph between velocity and acceleration of a particle executing S.H.M. can be
1. A circle
2. An ellipse
3. A straight line
4. Both (1) & (2)
The potential energy of a particle of mass m executing SHM is given by U = A(1 - cos2x), where x is the instantaneous displacement of the particle. The time period of oscillation is
1. π√mA
2. 2π√mA
3. π√m2A
4. 2π√m2A
A uniform rod of length l is suspended at l4 from one end and made to undergo small oscillations. The time period of oscillation is:
1. 2π√7l12g
2. 2π√3l7g
3. 2π√7l3g
4. 2π√4l5g
A simple pendulum with a metallic bob has a time period T. The bob is now immersed in a nonviscous liquid and the time period is found to be √5T. The ratio of the density of the metal to that of liquid is
1. 1/4
2. 4/3
3. 5/4
4. 7/3
A particle executes SHM with time period T. The time period of oscillation of total energy is:
1. T
2. 2T
3. T2
4. Infinite
A particle is executing linear simple harmonic motion with an amplitude a and an angular frequency ω. Its average speed for its motion from extreme to mean position will be:
1. aω4
2. aω2π
3. 2aωπ
4. aω√3π








