The equation of a SHM is given as $\mathrm{x} = 5\sin \left(4\mathrm{\pi t} + \frac{\mathrm{\pi }}{3}\right)$, where \(\mathrm t\) is in seconds and \(\mathrm x\) in meters. During a complete cycle, the average speed of the oscillator is:
1. zero
2. \(10\) m/s 
3. \(20\) m/s
4. \(40\) m/s

The equation of a simple harmonic oscillator is given as $\mathrm{y} = \mathrm{Asin}\left(20\mathrm{\pi t} + \frac{\mathrm{\pi }}{3}\right)$, where t is in seconds. The frequency with which kinetic energy oscillates is

1.  5 Hz

2.  10 Hz 

3.  20 Hz

4.  40 Hz

What is the period of oscillation of the block shown in the figure?

If a simple pendulum is brought deep inside a mine from the earth's surface, its time period of oscillation will:

The amplitude of a simple harmonic oscillator is A and speed at the mean position is ${\mathrm{v}}_0$. The speed of the oscillator at the position $\mathrm{x} = \frac{\mathrm{A}}{\sqrt{3}}$ is:

1.  $\frac{2v_0}{\sqrt{3}}$

2.  $\frac{\sqrt{2}{v}_0}{3}$

3.  $\frac{2}{3}{v}_0$

4.  $\frac{\sqrt{2}{v}_0}{\sqrt{3}}$

The initial phase of the particle executing SHM with y = 4 sin $\mathrm{\omega }$t + 3 cos $\mathrm{\omega }$t is 

1.  53°

2.  37° 

3.  90°

4.  45°

A spring-block system is brought from the Earth's surface to deep inside the mine. Its period of oscillation will:

A particle executes S.H.M with amplitude A. If the time taken by the particle to travel from -A to A/2 is 4 seconds, its time period is 

1.  4s

2.  8s 

3.  12 s

4.  18 s

Two simple harmonic motions are represented by ${\mathrm{y}}_1 = 6 \sin \left(2\mathrm{\pi t} + \frac{\mathrm{\pi }}{3}\right)$ and ${\mathrm{y}}_2 = 3\left(\sin 2\mathrm{\pi t} + \cos 2\mathrm{\pi t}\right)$. The ratio of their amplitudes is

1.  $\sqrt{2}$

2.  $\frac{2}{3}$

3.  $\frac{1}{2}$

4.  $2\sqrt{2}$

A disc executes S.H.M. about the axis XX' in the plane of the disc as shown in the figure. Its time period of oscillation is:

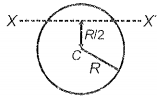
1.  $\mathrm{\pi }\sqrt{\frac{6\mathrm{R}}{\mathrm{g}}}$

2.  $2\mathrm{\pi }\sqrt{\frac{\mathrm{R}}{\mathrm{g}}}$

3.  $2\mathrm{\pi }\sqrt{\frac{2\mathrm{R}}{\mathrm{g}}}$

4.  $\mathrm{\pi }\sqrt{\frac{3\mathrm{R}}{2\mathrm{g}}}$