A body of mass m hanging with the help of three springs, each of spring constant k as shown. If the mass is slightly displaced and released, then the system will oscillate with the time period
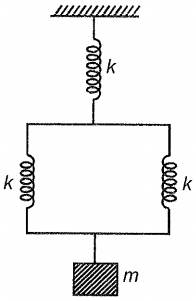
1. 2π√m3k
2. 2π√2m3k
3. 2π√3m2k
4. 2π√3mk

A block of mass m attached to a spring of constant k oscillates on a smooth surface. The other end of the spring is fixed to a wall. When spring is at its natural length, the speed of the block is v. Displacement of the block from its mean position before coming to instantaneous rest is:
1. √mvk
2. v√mk
3. m√vk
4. 1k√mv
A particle under SHM has a maximum speed of 30 cm/s and a maximum acceleration of 60 cm/s? The time period of oscillation (in second) is
1. π2
2. 2π
3. π
4. 4π
The x-t graph of a particle undergoing SHM is shown below. The acceleration of the particle at t = 43 is:
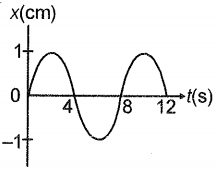
1. √332π3 cm/s2
2. -π232 cm/s2
3. π232 cm/s2
4. -√332π2 cm/s2
The position-time (y - t) graph of a particle executing S.H.M. is shown. The time period of the particle is 4 seconds. Equation of particle executing S.H.M. is
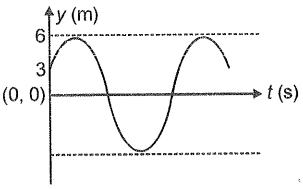
1. y = 6 sin(2πt + π6)
2. y = 6 sin(π2t + π3)
3. y = 6 sin(π2t + π6)
4. y = 6 sin(π2t - π6)
A particle starts executing SHM from an extreme position with time period T and amplitude A. The distance travelled by the particle in time 15T18is:
1. 3.5 A
2. 2.5 A
3. 0.5 A
4. 1.5
Two tunnels are dug across the earth as shown in the figure. Balls A and B are dropped in the tunnels. If the time period of oscillation of ball A is T, the time period of the oscillation of ball B is :
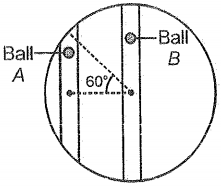
1. T
2. √32T
3. 2√3T
4. 23T
Select the correct statement(s) regarding S.H.M.
1. The average speed of particle executing S.H.M. is zero in one complete oscillation.
2. The graph between the kinetic and potential energy of a particle executing S.H.M. is a straight line.
3. Restoring force acting on the particle is directed towards a fixed point and is directly proportional to the displacement from the mean position of the particle.
4. Both (2) & (3)
The graph between the velocity (v) of a particle executing SHM and its displacement (x) is shown in the figure. The time period of oscillation for this SHM will be:
1. √αβ
2. 2π√αβ
3. 2π(βα)
4. 2π(αβ)
The displacement of a body performing simple harmonic motion is represented by x = asin(2πt + π3). After what approximate time from t = 0, the speed of the particle becomes maximum for the first time?
1. 0.12 s
2. 0.24 s
3. 0.33 s
4. 0.42 s







