Instantaneous acceleration (in ms-2) of a particle executing S.H.M. is given by a = -π4sin(π4t - π6). The maximum speed of the particle will occur first time at
1. 1.75 s
2. 1.4 s
3. 1.2 s
4. 0.67 s
A particle moves according to the equation x = Acosπ2t. Distance covered by it in the time interval of t =0 to t =3 s is: (symbols have their usual meanings)
1. A
2. 4A
3. 3A
4. 2A
1. 3T2
2. 3T4
3. 2T3
4. 4T3
A body of mass m hanging with the help of three springs, each of spring constant k as shown. If the mass is slightly displaced and released, then the system will oscillate with the time period
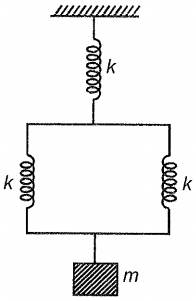
1. 2π√m3k
2. 2π√2m3k
3. 2π√3m2k
4. 2π√3mk
A block of mass m attached to a spring of constant k oscillates on a smooth surface. The other end of the spring is fixed to a wall. When spring is at its natural length, the speed of the block is v. Displacement of the block from its mean position before coming to instantaneous rest is:
1. √mvk
2. v√mk
3. m√vk
4. 1k√mv
A particle under SHM has a maximum speed of 30 cm/s and a maximum acceleration of 60 cm/s? The time period of oscillation (in second) is
1. π2
2. 2π
3. π
4. 4π
The x-t graph of a particle undergoing SHM is shown below. The acceleration of the particle at t = 43 is:
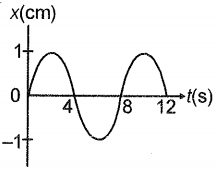
1. √332π3 cm/s2
2. -π232 cm/s2
3. π232 cm/s2
4. -√332π2 cm/s2
The position-time (y - t) graph of a particle executing S.H.M. is shown. The time period of the particle is 4 seconds. Equation of particle executing S.H.M. is
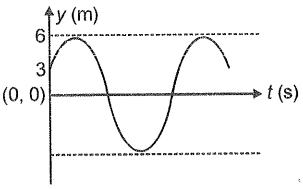
1. y = 6 sin(2πt + π6)
2. y = 6 sin(π2t + π3)
3. y = 6 sin(π2t + π6)
4. y = 6 sin(π2t - π6)
A particle starts executing SHM from an extreme position with time period T and amplitude A. The distance travelled by the particle in time 15T18is:
1. 3.5 A
2. 2.5 A
3. 0.5 A
4. 1.5
Two tunnels are dug across the earth as shown in the figure. Balls A and B are dropped in the tunnels. If the time period of oscillation of ball A is T, the time period of the oscillation of ball B is :
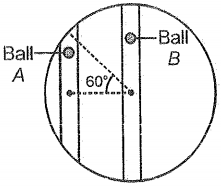
1. T
2. √32T
3. 2√3T
4. 23T






