The time period of the given spring-mass system is:

              

1.	\(2\pi \sqrt{\dfrac{m}{k}}\)	2.	\(2\pi \sqrt{\dfrac{m}{2k}}\)
3.	\(2\pi \sqrt{\dfrac{2m}{\sqrt{3}k}}\)	4.	\(\pi \sqrt{\dfrac{m}{k}}\)

The equation of simple harmonic motion is given by X = (4 cm)$\sin \left(6.28 t + \frac{5\mathrm{\pi }}{3}\right)$, then maximum velocity of the particle in simple harmonic motion is: 

1.  25.12 m/s 

2.  25.12 cm/s 

3.  12.56 m/s 

4.  12.56 cm/s

A spring pendulum is placed on a rotating table. The initial angular velocity of the table is \(\omega_{0}\) and the time period of the pendulum is \(T_{0}.\) If the the angular velocity of the table becomes \(2\omega_{0},\) then the new time period of the pendulum will be:
1. \(2T_{0}\)
2. \(T_0\sqrt{2}\)
3. the same
4. \(\dfrac{T_0}{\sqrt{2}}\)

If the vertical spring-mass system is dipped in a non-viscous liquid, then:

The displacement \( x\) of a particle varies with time \(t\) as \(x = A sin\left (\frac{2\pi t}{T} +\frac{\pi}{3} \right)\)$\mathrm{}$. The time taken by the particle to reach from \(x = \frac{A}{2} \) to \(x = -\frac{A}{2} \) will be:

Force on a particle \(F\) varies with time \(t\) as shown in the given graph. The displacement \(x\) vs time \(t\) graph corresponding to the force-time graph will be:
          

1.		2.
3.		4.

The time period of a simple pendulum in a stationary trolley is \(T_1.\) If the trolley is moving with a constant speed, then the time period is \(T_2.\) Then: 
1. \(T_1>T _2\)
2. \(T_1<T _2\)
3. \(T_1=T _2\)
4. \(T_2= \infty \)

 A particle is moving along the x-axis. The speed of particle v varies with position x as $\frac{{\mathrm{v}}^2}{144} + \frac{{\mathrm{x}}^2}{9} = 1$. The time period of S.H.M is

1.  $\mathrm{\pi } \mathrm{unit}$

2.  $\frac{3\mathrm{\pi }}{2} \mathrm{unit}$

3.  $\frac{\mathrm{\pi }}{2} \mathrm{unit}$

4.  $\frac{\mathrm{\pi }}{4} \mathrm{unit}$

A block of mass m is attached to a massless spring having a spring constant k. The other end of the spring is fixed from the wall of a trolley, as shown in the figure. Spring is initially unstretched and the trolley starts moving toward the direction shown. Its velocity-time graph is also shown.

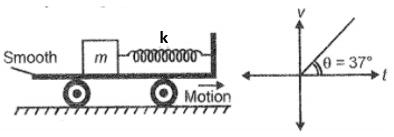
The energy of oscillation, as seen from the trolley is:

1.  $\frac{32{\mathrm{m}}^2}{6\mathrm{k}}$

2.  $\frac{9{\mathrm{m}}^2}{8\mathrm{k}}$

3.  $\frac{9{\mathrm{m}}^2}{32\mathrm{k}}$

4.  $\frac{8{\mathrm{m}}^2}{9\mathrm{k}}$