A body is projected with velocity 20√3 m/s with an angle of projection 60° with horizontal. Calculate velocity on that point where body makes an angle 30° with the horizontal.
1. 20 m/s
2. 20√3 m/s
3. 10√3 m/s
4. 10 m/s
In a uniform circular motion, which of the following quantity is not constant
1. Angular momentum
2. Speed
3. Kinetic energy
4. Momentum
A particle is moving with veocity →v=k(yˆi+xˆj); where k is constant. The general equation for the path is:
1. y=x2+constant
2. y2=x2+constant
3. y=x+constant
4. xy=constant
A particle is projected with a velocity u making an angle θ with the horizontal. At any instant, its velocity v is at right angles to its initial velocity u; then v is:
1. ucosθ
2. utanθ
3. ucotθ
4. usecθ
A projectile is given an initial velocity of ˆi+2ˆj. The cartesian equation of its path is (g = 10 ms-2)
1. y=2x-5x2
2. y=x-5x2
3. 4y=2x-5x2
4. y=2x-25x2
A ship A is moving westwards with a speed of 10 km h-1 and a ship B, 100 km south of A is moving northwards with a speed of 10 km h-1. The time after which the distance between them becomes the shortest, is:
1. 5 hr
2. 5√2 hr
3. 10√2 hr
4. 0 hr
Time taken by the projectile to reach from A to B is t. Then the distance AB is equal to :
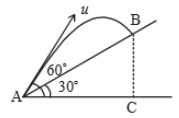

1. ut√3
2. √3ut2
3. √3ut
4. 2ut
A particle projected with kinetic energy k0 with an angle of projection θ. Then the variation of kinetic K with vertical displacement y is
1. linear
2. parabolic
3. hyperbolic
4. periodic
A river is flowing with a speed of 1 km/hr. A swimmer wants to go to point 'C' starting from 'A'. He swims with a speed of 5 km/hr, at an angle θ with respect to the river. If AB = BC = 400 m. Then

1. The time taken by the man is 12 min
2. The time taken by the man is 8 min
3. The value of θ is 45°
4. The value of θ is 53°
A body is thrown horizontally with a velocity √2gh from the top of a tower of height h. It strikes the level ground through the foot of the tower at a distance x from the tower. The value of x is:
| 1. | h | 2. | h2 |
| 3. | 2h | 4. | 2h3 |






