Ncert Exercises & Solutions

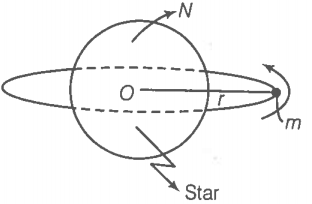
Q. 34 A star like the sun has several bodies moving around it at different distances. Consider that all of them are moving in circular orbits. Let r be the distance of a body from the centre of the star and let its linear velocity be v, angular velocity $ω$ , kinetic energy K, gravitational potential energy U, total energy E & and angular momentum L. As the radius r of the orbit increases, determine which of the above quantities increases and which one decreases.

Hint: The kinetic energy, potential energy and angular momentum vary with the distance of the body from the star.

Step 1: Find the kinetic energy and the potential energy of the body.

The situation is shown in the diagram where a body of mass m is revolving around a star of mass M.

The linear velocity of the body,

v = \sqrt{\frac{G M}{r}}

\Rightarrow v \propto \frac{1}{\sqrt{r}}

Therefore, when r increases, v decreases.
Angular velocity of the body,

ω = \frac{2 π}{k r^{3 / 2}} \Rightarrow ω \propto \frac{1}{r^{3 / 2}} (∵ ω = \frac{2 π}{T})

Therefore, when r increases,

ω

decreases.
The kinetic energy of the body, K

= \frac{1}{2} m v^{2} = \frac{1}{2} m \times \frac{G M}{r} = \frac{G M m}{2 r}

∴ K \propto \frac{1}{r}

Therefore, when r increases, KE decreases.
The gravitational potential energy of the body,

U = - \frac{G M m}{r} \Rightarrow U \propto - \frac{1}{r}

Therefore, when r increases, PE becomes less negative i.e., increases.

Step 2: Find the total energy of the body.

The total energy of the body,

E = K E + P E = \frac{G M m}{2 r} + (- \frac{G M m}{r}) = - \frac{G M m}{2 r}

Therefore, when r increases, the total energy becomes less negative i.e., increases.

Step 3: Find the angular momentum of the body.

Angular momentum of the body, L $= m v r = m r \sqrt{\frac{G M}{r}} = m \sqrt{G M r}$ $\Rightarrow L \propto \sqrt{r}$

Therefore, when r increases, angular momentum L increases.

NEET Information

courses

Company

Botany Questions

Chemistry Questions

Physics Questions

Zoology Questions