Let M and R be the mass and radius of the half-disc, mass per unit area of the half-disc

$\mathrm{m}=\frac{\mathrm{M}}{\frac{1}{2}{\mathrm{\pi R}}^2}=\frac{2\mathrm{M}}{{\mathrm{\pi R}}^2}$

Step 2: Find the position of center of mass of small element.

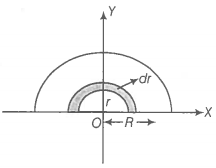
(a) The half-disc can be supposed to be consists of a large number of semicircular rings of mass dm and thickness d, and radii ranging from r = 0 to r = R.

The surface area of semicircular ring of radius r and of thickness $dr=\frac{1}{2}2\pi r\times dr=\pi rdr$
$\therefore$ Mass of this elementary ring, $dm=\pi rdr\times \frac{2M}{\pi R^2}$
$dm=\frac{2M}{R^2}rdr$
If (x, y) are coordinates of the center of mass of this element, then,
$\begin{array}{l}(x,y)=(0,\frac{2r}{\pi })\\ \mathit{\therefore }\mathit{ }x=0\text{ and }y=\frac{2r}{\pi }\end{array}$
Let ${\mathrm{x}}_{\mathrm{CM}}\text{ and }{\mathrm{y}}_{\mathrm{CM}}$ be the coordinates of the center of mass of the semicircular disc.

Then

Step 3: Find the position of center of mass for half-disc.

$\begin{array}{l}{\mathrm{x}}_{\mathrm{CM}}=\frac{1}{\mathrm{M}}{\int }_0^{\mathrm{R}}\mathrm{xdm}=\frac{1}{\mathrm{M}}{\int }_0^{\mathrm{R}}0\mathrm{dm}=0\\ {\mathrm{y}}_{\mathrm{CM}}=\frac{1}{\mathrm{M}}{\int }_0^{\mathrm{R}}\mathrm{ydm}=\frac{1}{\mathrm{M}}{\int }_0^{\mathrm{R}}\frac{2\mathrm{r}}{\mathrm{\pi }}\times \left(\frac{2\mathrm{M}}{{\mathrm{R}}^2}\mathrm{rdr}\right)\\ =\frac{4}{{\mathrm{\pi R}}^2}{\int }_0^{\mathrm{R}}{\mathrm{r}}^2\mathrm{dr}=\frac{4}{{\mathrm{\pi R}}^2}[\frac{{\mathrm{r}}^3}{3}{]}_0^{\mathrm{R}}\\ =\frac{4}{{\mathrm{\pi R}}^2}\times (\frac{{\mathrm{R}}^3}{3}-0)=\frac{4\mathrm{R}}{3\mathrm{\pi }}\end{array}$

$\therefore \text{ Centre of mass of the semicircular disc }=(0,\frac{4\mathrm{R}}{3\mathrm{\pi }})$

(b)

Step 4: Find centre of mass of a uniform quarter disc.

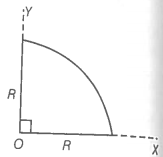
using symmetry
For a half-disc along the y-axis center of mass be at $\mathrm{x}=\frac{4\mathrm{R}}{3\mathrm{\pi }}$
For a half-disc along the x-axis center of mass will be at $\mathrm{x}=\frac{4\mathrm{R}}{3\mathrm{\pi }}$
Hence, for the quarter disc center of mass $=\left(\frac{4\mathrm{R}}{3\mathrm{\pi }},\frac{4\mathrm{R}}{3\mathrm{\pi }}\right)$