Question 7. 21. A solid cylinder rolls up an inclined plane of angle of inclination 30°. At the bottom of the inclined plane, the centre of mass of the cylinder has a speed of 5 m/s.
(a) How far will the cylinder go up the plane?
(b) How long will it take to return to the bottom?
Velocity of the solid cylinder, v = 5 m/s
Angle of inclination, θ = 30°
Height reached by the cylinder =h
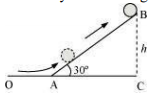
Energy of the cylinder at point A:
KErot=KE trans 12Iω2=12mv2
Energy of the cylinder at point B = mgh
Using the law of conservation of energy,
12Iω2+12mv2=mgh
⇒ 12(12mr2)ω2+12mv2=mgh=14mr2ω2+12mv2=mgh14v2+12v2=gh (as, v=rω)34v2=gh⇒h=34v2g=34×5×59.8=1.91m
In ΔABC:
sinθ=BCABsin30∘=hABAB=1.910.5=3.82m
Hence, the cylinder will go 3.82 m up the inclined plane.
(b) For a radius of gyration K, the velocity of the cylinder at the instance when it rolls back to the bottom is,
v=(2gh1+K2R2)12⇒ v=(2gABsinθ1+K2R2)12 For the solid cylinder, K2=R22
⇒ v=(2gABsinθ1+12)12=(43gABsinθ)12
The time taken to return to the bottom is,
t=ABv=AB(43gABsinθ)12=(3AB4gsinθ)12=(11.4619.6)12=0.764s
Therefore, the total time taken by the cylinder to return to the bottom=2×0.764 =1.53 s

© 2025 GoodEd Technologies Pvt. Ltd.
