A ball of mass m, moving with a speed , collide inelastically (e > 0) with an identical ball at rest. Show that
(a) For head-on collision, both the balls move forward.
(b) For a general collision, the angle between the two velocities of scattered balls is less than 90.
(a) Let are velocities of the two balls after the collision.
Now bv the Principle of conservation of linear momentum.
Since , has the same sign as , therefore, the ball moves on after collision.
Step 2: Interpret the (b) part.
(b) Consider the diagram below for a general collision.
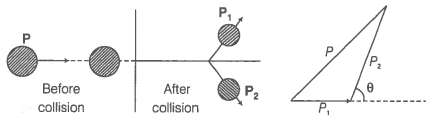
By the principle of conservation of linear momentum,
For inelastic collision, some KE is lost, hence
Thus, are related as shown in the figure.

© 2024 GoodEd Technologies Pvt. Ltd.