Solved Problems: Set 1
12.5 The Line Spectra of the Hydrogen Atom
According to the third postulate of Bohr’s model, when an atom makes a transition from the higher energy state with quantum number ni to the lower energy state with quantum number nf (nf < ni), the difference of energy is carried away by a photon of frequency νif such that
hνif = Eni – Enf (12.20)
Using Eq. (12.16), for Enf and Eni, we get
hνif = 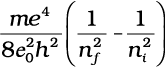 (12.21)
(12.21)
or νif = 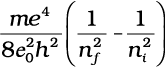 (12.22)
(12.22)
Equation (12.21) is the Rydberg formula, for the spectrum of the hydrogen atom. In this relation, if we take nf = 2 and ni = 3, 4, 5..., it reduces to a form similar to Eq. (12.10) for the Balmer series. The Rydberg constant R is readily identified to be
R =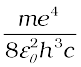 (12.23)
(12.23)
If we insert the values of various constants in Eq. (12.23), we get
R = 1.03 × 107 m–1
This is a value very close to the value (1.097 × 107 m–1) obtained from the empirical Balmer formula. This agreement between the theoretical and experimental values of the Rydberg constant provided a direct and striking confirmation of the Bohr’s model.
Since both nf and ni are integers, this immediately shows that in transitions between different atomic levels, light is radiated in various discrete frequencies. For hydrogen spectrum, the Balmer formula corresponds to nf = 2 and ni = 3, 4, 5, etc. The results of the Bohr’s model suggested the presence of other series spectra for hydrogen atom–those corresponding to transitions resulting from nf = 1 and ni = 2, 3, etc.; nf = 3 and ni = 4, 5, etc., and so on. Such series were identified in the course of spectroscopic investigations and are known as the Lyman, Balmer, Paschen, Brackett, and Pfund series. The electronic transitions corresponding to these series are shown in Fig. 12.9.
NEETprep Audio Note:
The explanation of the hydrogen atom spectrum provided by Bohr’s model was a brilliant achievement, which greatly stimulated progress towards the modern quantum theory. In 1922, Bohr was awarded Nobel Prize in Physics.
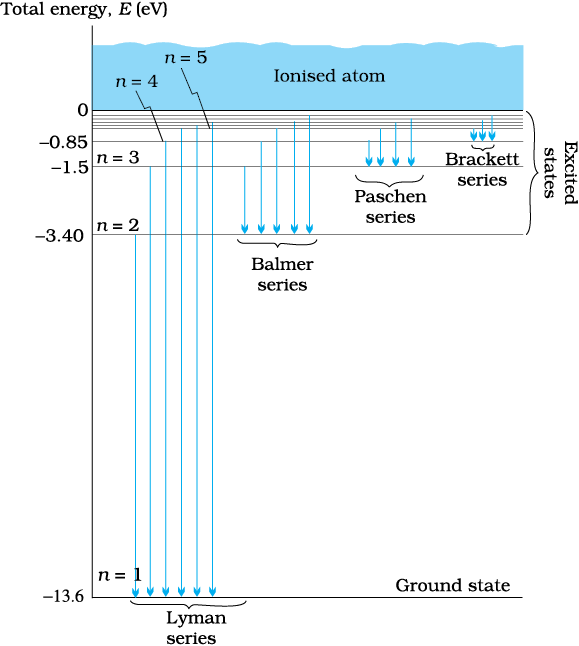
Figure 12.9 Line spectra originate in transitions between energy levels.
Example 12.6 Using the Rydberg formula, calculate the wavelengths of the first four spectral lines in the Lyman series of the hydrogen spectrum.
Solution The Rydberg formula is
hc/λif = 
The wavelengths of the first four lines in the Lyman series correspond to transitions from ni = 2,3,4,5 to nf = 1. We know that
 = 13.6 eV = 21.76 ×10–19 J
= 13.6 eV = 21.76 ×10–19 J
Therefore,
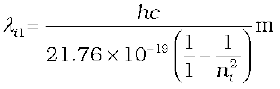
= 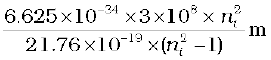 =
= 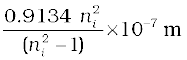
= 913.4 ni2/(ni2 –1) Å
Substituting ni = 2,3,4,5, we get λ21 = 1218 Å, λ31 = 1028 Å, λ41 = 974.3 Å, and λ51 = 951.4 Å.