Energy of Particle & de Broglie Wavelength
11.7 Particle Nature of Light: The Photon
Photoelectric effect thus gave evidence to the strange fact that light in interaction with matter behaved as if it was made of quanta or packets of energy, each of energy h ν.
Is the light quantum of energy to be associated with a particle? Einstein arrived at the important result, that the light quantum can also be associated with momentum (h ν/c). A definite value of energy as well as momentum is a strong sign that the light quantum can be associated with a particle. This particle was later named photon. The particle-like behaviour of light was further confirmed, in 1924, by the experiment of A.H. Compton (1892-1962) on scattering of X-rays from electrons. In 1921, Einstein was awarded the Nobel Prize in Physics for his contribution to theoretical physics and the photoelectric effect. In 1923, Millikan was awarded the Nobel Prize in physics for his work on the elementary charge of electricity and on the photoelectric effect.
We can summarise the photon picture of electromagnetic radiation as follows:
(i) In interaction of radiation with matter, radiation behaves as if it is made up of particles called photons.
(ii) Each photon has energy E (=hν) and momentum p (= h ν/c), and speed c, the speed of light.
(iii) All photons of light of a particular frequency ν, or wavelength λ, have the same energy E (=hν = hc/λ) and momentum p (= hν/c= h/λ), whatever the intensity of radiation may be. By increasing the intensity of light of given wavelength, there is only an increase in the number of photons per second crossing a given area, with each photon having the same energy. Thus, photon energy is independent of intensity of radiation.
(iv) Photons are electrically neutral and are not deflected by electric and magnetic fields.
(v) In a photon-particle collision (such as photon-electron collision), the total energy and total momentum are conserved. However, the number of photons may not be conserved in a collision. The photon may be absorbed or a new photon may be created.
Example 11.1 Monochromatic light of frequency 6.0 ×1014 Hz is produced by a laser. The power emitted is 2.0 ×10–3 W. (a) What is the energy of a photon in the light beam? (b) How many photons per second, on an average, are emitted by the source?
Solution
(a) Each photon has an energy
E = h ν = ( 6.63 ×10–34 J s) (6.0 ×1014 Hz)
= 3.98 × 10–19 J
(b) If N is the number of photons emitted by the source per second, the power P transmitted in the beam equals N times the energy per photon E, so that P = N E. Then
N =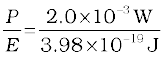 
= 5.0 ×1015 photons per second.
Example 11.2 The work function of caesium is 2.14 eV. Find (a) the threshold frequency for caesium, and (b) the wavelength of the incident light if the photocurrent is brought to zero by a stopping potential of 0.60 V.
Solution
(a) For the cut-off or threshold frequency, the energy h ν0 of the incident radiation must be equal to work function φ0, so that
ν0 = 
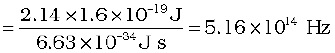
Thus, for frequencies less than this threshold frequency, no photoelectrons are ejected.
(b) Photocurrent reduces to zero, when maximum kinetic energy of the emitted photoelectrons equals the potential energy e V0 by the retarding potential V0. Einstein’s Photoelectric equation is
eV0 = hν 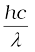– φ0 = 
– φ0
or, λ = hc/(eV0 + φ0)
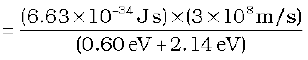
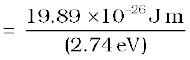
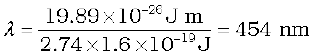
Example 11.3 The wavelength of light in the visible region is about 390 nm for violet colour, about 550 nm (average wavelength) for yellow-green colour and about 760 nm for red colour.
(a) What are the energies of photons in (eV) at the (i) violet end, (ii) average wavelength, yellow-green colour, and (iii) red end of the visible spectrum? (Take h = 6.63×10–34 J s and 1 eV = 1.6×10 –19J.)
(b) From which of the photosensitive materials with work functions listed in Table 11.1 and using the results of (i), (ii) and (iii) of (a), can you build a photoelectric device that operates with visible light?
Solution
(a) Energy of the incident photon, E = hν = hc/λ
E = (6.63×10–34J s) (3×108 m/s)/λ
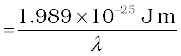
(i) For violet light, λ1 = 390 nm (lower wavelength end)
Incident photon energy, E1 =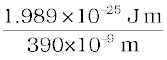 
= 5.10 × 10–19J
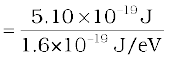= 3.19 eV
(ii) For yellow-green light, λ2 = 550 nm (average wavelength)
Incident photon energy, E2 =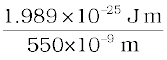 
= 3.62×10–19 J = 2.26 eV
(iii) For red light, λ3 = 760 nm (higher wavelength end)
Incident photon energy, E3 = 
= 2.62×10–19 J = 1.64 eV
(b) For a photoelectric device to operate, we require incident light energy E to be equal to or greater than the work function φ0 of the material. Thus, the photoelectric device will operate with violet light (with
E = 3.19 eV) photosensitive material Na (with φ0 = 2.75 eV), K (with
φ0 = 2.30 eV) and Cs (with φ0 = 2.14 eV). It will also operate with yellow-green light (with E = 2.26 eV) for Cs (with φ0 = 2.14 eV) only. However, it will not operate with red light (with E = 1.64 eV) for any of these photosensitive materials.